The High Frequency Testing of Vascular Grafts and Vascular Stents: Influence…

Stent Testing

The High Frequency Testing of Vascular Grafts and Vascular Stents: Influence of Sample Dimensions of Maximum Allowable Frequency

Biomedical Science Instrumentation, 35, pp. 339-346, (1999)

J.C. Conti¹, E.R. Strope, K.S. Price, L.M. Goldenberg

Dynatek Dalta Scientific Instruments, P.O. Box 254, Galena, MO 65656

1 Southwest Missouri State University, Dept. of Physics and Astronomy, 901 S. National Avenue, Springfield, MO 65804-0094

RMBS 1999

Keywords

Compliance, grafts, frequency, radial, testing, accelerated, durability, fatigue, stents, catheters

Abstract

There are over four dozen companies developing new products for the lucrative vascular graft and vascular stent markets. We have been studying the procedures used to pre-test vascular grafts, vascular stents, and mock arteries into which vascular stents are placed, so that appropriate high frequency durability/fatigue studies can be done in as short a time as possible, but also in a manner in which the data are highly reliable and dependable. In the past, we have evaluated the testing of the natural frequency response of the grafts, mock arteries, and stents in order to determine the frequencies that can be used for long-term life testing. In this paper, we present experiments that evaluate how product geometry affects product frequency response at various loading pressures. Results show that changing the dimensions of the device to be tested such that less fluid has to be injected (in the case of hydrodynamically driven experiments) results in the ability to test these products at a higher frequency.

Introduction

Radial compliance of a tube or vessel is defined as the relationship between the radius of a tube and the pressure inside that tube. Although there is general agreement on the definition of compliance, there have been a variety of techniques used to evaluate this parameter. Since most of the initial interest in the radial compliance of tubes was generated because of a concern relating to the long-term patency of vascular grafts [1-6], it has been postulated that it is the internal change in radius that most significantly affects the blood flow disturbance or the interaction of a graft or synthetic tube with an indwelling stent or catheter. A review of the literature indicates large variations, not only in the estimation of in vivo compliance, but also in the experimental designs used to evaluate compliance of both natural vessels and vascular grafts. Some of the work was done on excised vessels [7-8] or natural vessels that were exposed to air [9-10]. Many of the previous techniques utilized X-rays, and some took advantage of cantilevered beams [11-13] touching the vessel or vascular graft in order to determine the change in external diameter versus pressure. Additional experiments reported using various video techniques [14-19]. More recent investigations evaluating mechanical compliance found that the three-dimensional compliance of vascular grafts and mock latex arteries was dependent upon pulsation rate or testing frequency [20-22]. This knowledge directly affects the design of reliable protocols. There is, of course, a desire to acquire information about the durability or fatigue resistance of compliant products in a time period as short as possible. Consideration of the frequency response of the product under test as well as the time dependent characteristics of other experimental materials used, such as synthetic arteries housing vascular stents, becomes extremely important. Accelerated testing is plagued by problems, not just in the mechanical engineering area, but also in metal fatigue analysis and radiation of polymers. For example, increasing the dose of radiation or the storage temperature are common types of accelerated testing. While informative, these types of experiments, as well as all accelerated tests, are never perfect in design or concept. The reason, of course, is that high levels of exposure to an experimental variable, whether it is temperature, radiation, or pressure versus time, can never exactly replicate the real time situation. Experts in accelerated testing know this and take special precautions to evaluate what impact the unnatural stressing or loading has on the product’s ability to respond. In mechanical testing particularly, it is important to spend sufficient time on initial evaluation of the frequency response of all components before finalizing test protocols. As has been shown in vascular graft and mock artery testing at high frequencies, one needs to be concerned with how the motion of the graft or the mock artery containing the stent or catheter responds to increasing rates of testing [21-22]. The key to understanding these frequency dependent changes lies with consideration of the materials as well as the limitations of the instrumentation used during the accelerated testing. It is clearly the goal of long-term durability testing to evaluate properly the fatigue resistance of the product, and to do it in a time frame that is as short as possible. This assists early detection of design flaws, the schedule of product development personnel, and the rapid submission of products to regulatory agencies.

The general goal of accelerated testing should be to neither overload nor underload the product. Overloading can lead to unpredicted and unjustified product failure and may result in the tendency to ignore failures that occur, with potentially disastrous results. On the other hand, understressed products induce unjustified confidence in the long-term durability of the devices and may result in in vivo failures with equally disastrous results. A recent paper indicated some of the errors to avoid when monitoring the high speed testing of vascular grafts or synthetic arteries that contain medical products such as stents, stent grafts or catheters [23].

Thorough knowledge of accelerated durability testing of tubular elements allows modification of specific experimental variables and test design in order to test at higher speeds without jeopardizing the validity of experimental protocols and results.

General Considerations

The discussion will focus primarily on experiments carried out on precision built latex tubing or mock arteries. These are samples that are dipped on precisely ground mandrels with wall thicknesses accurate to 0.001 inch. Such precision allows replication of the three-dimensional properties of human vascular vessels. This is very important in protocols designed to evaluate the influence of indwelling devices on radial compliance of the vessels into which they are deployed. These mock arteries can also be used to evaluate bend and drag properties of catheters since the radial compliance and durometer of these vessels closely replicate that of natural vessels. Finally, they allow evaluation of the long-term durability and fatigue of products designed to remain indwelling in the vascular or cardiovascular system such as stents, grafts, and intraaortic balloon pumps.

In evaluating published techniques that greatly increase the testing frequency for high speed durability evaluations, two criteria must be examined. First, the frequency response of the latex tube into which the product is going to be delivered must be such that a pulsating load of 120/80mm Hg delivered at high speeds results in the same geometric excursion of the wall per cycle as it does when delivered at 60 or 70bpm. If this can be proven, then the validity of the accelerated test has passed one major criterion. Second, the product itself must have the ability to respond to the rapidly cycling stresses that are delivered with a particular experiment or protocol. Some products such as intraaortic balloon pumps, artificial hearts, and biological vascular grafts do represent the rate limiting factor in the design of experimental protocols. Vascular stents, on the other hand, are usually made of a high tensile metal material that usually can respond to high frequency stresses at least as well as the viscoelastic such as the mock arteries.

Over the years it has become common to test 10cm long vascular grafts and to sample along the manufacturing run or mandrel such that the testing is not sensitive to manufacturing position or time of acquisition. This has resulted in commonly adopting the 10cm length when evaluating vascular stents. In fact, initial experiments often saw more than one stent placed in a tube in order to use up the tube length efficiently. The placement was usually such that several diameters of length were allowed between the mounting position of the manifold and the position of the first stent, and several diameters in between stents. This approach led to some concern about migration of the stents within the tube and has, in general, been dropped. However, reconsideration of the 10cm length of the testing sample might be appropriate since this could likely change the frequency response of the viscoelastic items and allow increased speed of test. This represents one of the most ideal ways to increase the speed of testing of vascular grafts as well as synthetic arteries that contain medical devices. It would not require any assumptions concerning the effect of overstressing or overloading associated with very high speed testing. The explanation is as follows: If one increases the frequency of loading of a vascular graft that has a radial compliance of 5% at 72bpm, then at some frequency the apparent compliance of the graft will suddenly begin to drop. This represents the point at which the frequency of the pulsations in the graft are outrunning the ability of the graft wall to respond to the cyclic pressurization. It has been suggested, although not widely accepted by experts in the field of accelerated testing, that simply overpressurizing these grafts so as to give the same apparent change in geometry per cycle would allow testing at high speed. Take the example of a graft, tested at 120/80mm Hg, whose compliance drops from 6% at 72bpm to 2% at 2000bpm. If that same graft were tested at 540/240mm Hg at 2000bpm, it could be viewed as having the same geometric change per cycle as the 120/80 testing at 72bpm. The concern is the need to make assumptions about the change in pressure versus time and, therefore, the stress rates or strain rates of the polymer materials in these grafts. Certainly stress rates and strain rates are of concern with any accelerated testing. However, when dramatic overpressurization is necessary, the characteristics of this experimental system must be investigated much more thoroughly. The use of shorter lengths requires no assumptions and can allow reduced testing time without any trade-off in experimental reliability.

The second experiment was similar to the first except that multiple tubes were tested in each experimental situation and the sequence of the testing was different than the first experiment. Once the tube was mounted on the dynamic compliance tester, it was evaluated at all the speeds using the same pressure differential. In other words, the first experiment was carried out at 140/80mm Hg at 72bpm, the second at 140/80mm Hg at 800bpm, the third at 1200bpm, and so on. Then, the tubes were evaluated at 180/80mm Hg at increasing speeds. This allowed us to ascertain whether or not the sequence of the experiments had any influence on the resulting mechanical properties of the mock arteries. This is important to know because stressing a viscoelastic sample can have an influence on the mechanical properties and, therefore, a proper testing sequence must be carried out.

Results

Table 1 is a summary of the testing performed such that both pressures were completed before the speed of the instrument was increased. Comparing line 1 with line 3 at 72bpm, note that the 0-80mm Hg compliance is the same even though the second experiment extended over a greater pressure range. The radial compliance of the 80-140 (line 2) and 80-180 experiments (line 4) show somewhat different results (5.36 versus 5.67). Scanning across line 2 and line 4 in this initial column of data, notice that the radial compliance begins to drop with increasing testing frequency. Lines 1-8 represent the experiments carried out on 4cm long grafts, lines 9-12 on the 7cm long grafts, and lines 13-16 on the 10cm long grafts. In general, a 15% change in compliance is allowed before concerns arise about the effect of increased frequency. This would represent a 1% change in actual radial compliance with increasing speed. As seen on lines 14 and 16 with the10cm long samples, the 140/80 sample has experienced a 1% change in compliance by the time it reaches 800bpm, whereas the 180/80 reaches somewhere between 800 and 1200bpm before it begins to stiffen up beyond acceptable limits. In the results of the 7cm long samples, lines 10 and 12, the testing speed can reach nearly 1600bpm before the same reduction in compliance is obtained. Lines 6 and 8 show that testing can be carried out to nearly 2200bpm before attaining the 1% change in compliance from what was obtained at 72bpm, although there are some differences in the 140/80 and the 180/80 compliances. (Note: These compliances are normalized to a 100mm Hg differential.) This difference is easily explained by looking at Figures 1 and 2 which are the pressure-volume traces for the 140/80 and 180/80 experiments, respectively. The gradual flattening of the higher pressure trace (Figure 2) indicates higher compliance.

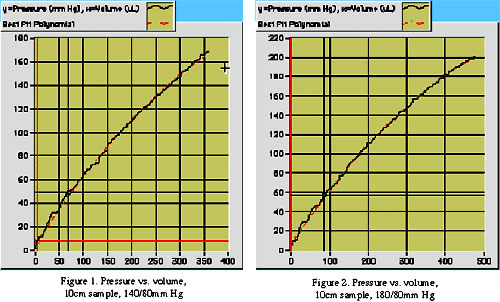
Table 2 is a summary of the data obtained for the more extensive experimentation with three samples for each data point. Very similar to the results found in the first experiment shown in Table 1, the compliances are all slightly higher than the 180/80 experiment versus the 140/80. Again, this has to do with a flattening of the curve as it approaches the 180mm Hg. But because the results between the 140/80 and 180/80 experiments are similar in both Tables 1 and 2, the conclusion is that the exact sequence of the testing (that is, sequencing the speed in Table 2 versus sequencing the pressure in Table 1) shows no significant difference and, for these short term experiments at least, is not an important variable.

Table 2 contains the more comprehensive experiments and includes standard deviations. Note that the standard deviations of these experiments are quite good and allow confidence to the first decimal place in these radial compliance measurements. Experience over the past twenty years with different internal and external compliance techniques often yielded much less accurate and precise results.

As in the evaluation of the first experiment in Table 1, the greatest concern is a 15% reduction in compliance as the frequency of the testing increases. Lines 33-40 present results for the 10cm long mock arteries. As shown on lines 34 and 36, attaining a frequency of 1200bpm causes a large enough reduction in the compliance to render the speed too fast for accelerated testing.

The evaluation of the 7cm long samples (lines 25-32) indicates that testing can be successfully carried out up to and including 1200bpm. The higher pressure results are found on lines 26, 28, 30, and 32. This means that by changing the length of the sample from 10 to 7cm, testing speed can increase by 400bpm.

When reducing the length of the sample down to 4cm, the compliance change of the 180/80 samples when increasing from 72 to 1600bpm (lines 20 and 24) indicate that testing at 1600bpm would certainly be acceptable. In addition, these data show that testing as fast as 2000bpm would be acceptable if the testing excursion were only 140/80mm Hg, as indicated on lines 18 and 22.

Discussion

The purpose of this series of experiments is to determine whether or not it would be possible, by reducing the sample length, to test viscoelastic products that have mechanical properties similar to those of human arterial components at faster frequencies than would normally be allowed. The assumption that reducing the tube length would have an impact on maximum speed of test seems at first self evident. However, over the past two decades experience has shown that accelerated testing is not always straightforward. These experiments, however, have shown that a shortened sample length for both vascular grafts, as well as mock arteries into which medical products will be deployed, can have a substantial and important impact on the maximum allowable testing frequency.

The only caveat to this approach is that by using shorter samples, more samples from any particular lots or runs or mandrels must be tested in order to gain the same level of confidence as by testing 10cm long samples. In addition, more has to be done in evaluating the minimum lengths that are appropriate to account for defects, clamping, and full expansion of the whole sample per cycle.

We encourage our fellow scientists, engineers, and regulatory professionals to evaluate the use of shorter samples so that faster testing speeds can be attained using rational and reliable protocols rather than allowing testing professionals to be enticed into the method of overpressurization to justify very high speed testing.

References

1. Kinley, C. E., and Marble, A. E., Compliance. J. Cardiovasc. Surg., 21, 163-170 (1980).

2. Clark, R. E., Apostolou, S., and Kardos, J. L., Mismatch of Mechanical Properties as a Cause of Arterial Prosthesis Thrombosis. Surg. Forum, 27, 208-217 (1976).

3. Gonza, E. R., Mason, W. F., Marble, A. E., and others, Necessity for Elastic Properties in Synthetic Arterial Grafts. Can. J. Surg., 17, 176-179 (1974).

4. Lyman, D. J., Fazzio, F. J., Voorhees, H., and others, Compliance as a Factor Effecting the Patency of Copolyurethane Vascular Grafts. J.
Biomed. Master. Res., 12, 337-339 (1978).

5. Abbott, W. M., and Bouchier-Hayes, D. J., The Role of Mechanical Properties in Graft Design. In: Graft Materials in Vascular Surgery. Edited by H. Dardik. Miami: Symposia Specialists, Inc., 59 (1978).

6. Baird, R. N., Kidson, I. G., L’Italien, J. G., and Abbott, M. W., Dynamic Compliance of Arterial Grafts. Am. J. Physiol., 2, 568-572 (1977).

7. Bergel, D. H., The Static Elastic Properties of the Arterial Wall. J. Physiol. (London), 156, 445 (1961).

8. Bergel, D. H., The Dynamic Elastic Properties of the Arterial Wall. J. Physiol. (London), 156, 448 (1961).

9. Patel, D. J., De Freitan, F. M., Freenfield, J. R., and Fry, D. L., Relation of Radius to Pressure Along the Aorta in Living Dogs. J. Appl. Physiol, 18, 1111 (1963).

10. Paterson, L. H. Jensen, R. E. and Parnell, J., Mechanical Properties of Arteries In Vivo. Circulation Res., 8, 622 (1960).

11. Walden, R., L’Italien, G. J., Megerman, J., and Abbott, W. M., Matched Elastic Properties and Successful Arterial Grafting. Arch. Surg., 115, 1166-1169 (1980).

12. Gow, B. S., and Taylor, G., Measurement of Viscoelastic Properties of Arteries in the Living Dog, Circulatory Research, 23, 111 (1968).

13. Gow, B. S., An Electrical Caliper for the Measurement of Pulsatile Arterial Diameter In Vivo. J. Appl. Physiol, 21, 1122 (1960).

14. Gotoh, F., Muramatsu, F., Fukuuchi, Y., Okaysu, H., Tanaka, K., Suzuki, N., and Kobari, M., Video Camera Method for Simultaneous Measurement of Blood Flow Velocity and Pial Vessel Diameter. J. Cereb. Blood Flow Metab.,
2, 421-428 (1982).

15. Assmann, R. and Henrich, H., A Video-Angiometer for Simultaneous and Continuous Measurement of Inner and Outer Vessel Diameters. Pflugers Arch., 376, 263-266 (1978).

16. Halpern, W., Mongeon, S. A., and Root, D. T., Stress, Tension and Myogenic Aspects of Small Isolated Extraparenchymal Rat Arteries. In: Smooth Muscle Contraction, edited by N.L. Stephens. New York : Dekker, 427-456 (1984).

17. Intaglietta, M. and Tompkins, W. R., On-Line Measurement of Microvascular Dimensions by Television Microscopy. J. Appl. Physiol., 32, 546-551 (1972).

18. Wiederhielm, C. A., Continuous Recording of Arteriolar Dimensions with a Television Microscope. J. Appl. Physiol., 18, 1041-1042 (1963).

19. Halpern, W., Osol, G., Coy, G. S., Mechanical Behavior of Pressurized In Vitro Prearteriolar Vessels Determined with a Video System. Annals of Biomedical Engineering, 12, 463-479 (1984).

20. Conti, J. C., Strope, E. R., Rohde, D. R. and Greisler, H. P., A New Technique to Determine Vascular Compliance In Vivo, National Heart Lung and Blood Institute Contractors Meeting. Louisville , KY , 1989.

21. Bozzi, R., Conti, J. C., Soldani, G., Spence, L., Strope, E., and Withrow, D., Relating the Frequency Dependent Radial Compliance to the Tensile Modulus of Polyurethane and Latex Vascular Grafts. Transactions 5th World Biomaterials Congress, 434, U2 (1996).

22. Conti, J. C., Strope, E. R., Rohde, D. J., and Spence, L. D., Frequency Dependent Radial Compliance of Latex Tubing. Biomedical
Sciences Instrumentation, 33, 524-529 (1997).

23. Conti, J. C., Strope, E. R., Price, K. S., and Goldenberg, L. D., Sources of Error in Monitoring High Speed Testing of Vascular Grafts, Biomedical Sciences Instrumentation, 34, 240-245 (1998).