Frequency Dependent Radial Compliance of Latex Tubing
Mock Artery Compliance
by | RMBS 1997 | Publications, Compliance
Frequency Dependent Radial Compliance of Latex Tubing
Biomedical Science Instrumentation, 33, pp. 524-529, (1997)
J.C. CONTI,1 E.R. Strope, D.J. Rohde, L.D. Spence
Dynatek Dalta Scientific Instruments
PO Box 254, Galena, MO 65656
1 Department of Physics and Astronomy, Southwest Missouri State University
RMBS 1997
Keywords
Compliance, latex, tubing, mechanical, grafts, stent, artery, frequency, radial, testing
Abstract
Custom latex tubing is often used in medical device evaluation. Examples include thin-walled devices used to reduce leakage of porous vascular grafts, and thicker-walled prototypes used as mechanically equivalent synthetic arteries. Medical devices such as stents and balloons are introduced into these for mechanically comparable in vitro testing. The three-dimensional mechanical properties of these tubes are critically important, particularly in accelerated testing, since they are primarily designed to replicate the mechanical rather than biological properties of in vivo arteries.
This paper explores the instrumentation and protocols necessary to evaluate the frequency dependent radial compliance of precision built latex tubing. Five cm long samples of custom dipped latex tubing 6mm in saline for 48 or 96 hours before being mounted on a dynamic internal compliance tester. Each tube was tested initially at 70bpm to establish the internal radial compliance at the physiologically relevant rate. The frequency of the test was then increased incrementally and the radial compliance re-checked. In the most extreme case, tubes were tested up to 2700bpm. In each case, the volume, pressure, and length of the tube were monitored continuously.
The results show a complicated relationship between testing frequency and radial compliance.
Introduction
Radial, longitudinal, or volume compliance of a tube or vessel is defined as the relationship between the particular dimension or volumetric parameter and the pressure inside the tube. Although there is agreement on the definition of compliance, there have been a variety of techniques used to evaluate this parameter. during static or dynamic pulsing [9-10]. Many of these earlier tests were done on various biological specimens [11-14]. Greater understanding of the hydrodynamics of pulsatile flow have led some authors to utilize the arterial pulse wave velocities to analyze the distensibility of vessels [15-16]. More recently, several investigators have utilized pressure measurements combined with video imaging of in vivo and in vitro vessels to monitor the diameter and pressure relationships of vessels [17-22]. Although these are of great interest from an historical perspective, one problem common to all these techniques is the lack of measurement of the internal diameter of the tubes. It is clearly important to understand what the internal compliance of these vessels are because, when utilized in contact with blood, it is the position of the inside wall vs. time and not the outside wall vs. time that is important. This issue of internal vs. external compliance as well as the concern for dynamic vs. static testing was addressed in two recent publications [23-24]. The use of latex or other synthetic materials to fabricate tubes of known compliance has increased recently. One familiar use is to insert thin-walled tubes into porous vascular grafts so that the mechanical and fatigue properties of these grafts can be evaluated without excessive leakage. Another more recent use of these types of tubes is as synthetic arteries into which the current generation of intravascular stents are deployed. Stents can then be tested at real time or in an accelerated manner to evaluate their durability. Because these tubes can be used in accelerated or high speed cyclic testing, it is important to understand the mechanical properties of these vessels at the accelerated rates to which they will be exposed. It is the purpose of this paper to establish instrumentation and protocols that can be used to evaluate the radial compliance of various synthetic tubes at various frequencies and to determine the relationship between testing frequency and the compliance of latex tubes.
Materials and Methods
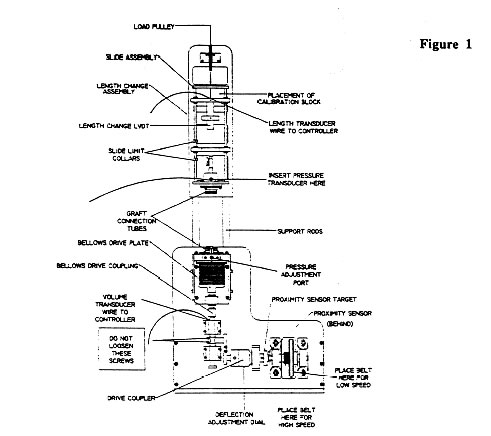
In order to accomplish the dynamic internal compliance measurements, a machine was modified that allowed for the mounting and internal pressurization of target latex tubes. See Figure 1. The device is made up of a cyclic drive system powered by an adjustable rate DC motor. The motor is attached to a volume injection system through a precision drive coupler (the head from a milling machine) and can be adjusted down to 0.001 inch. This drive coupler is attached to a push rod which is in turn attached to one side of a custom-built nickel bellows. Attached to the drive rod on the opposite side of the bellows is a linear voltage to displacement transducer (LVDT). By using a calibration step, the voltage output from this transducer can be directly converted to a volume ejected by the bellows. At the exit side of the bellows is the synthetic graft or sample connection tube. This allows for the quick placement or removal of subject experimental tubes. On the upper or distal connection tube is a hole that can be used to void air from the system and then to insert a high speed (80kHz) solid state pressure transducer. This upper graft connection/transducer adapter is the bottom section of a sliding upper assembly that, with the use of a balancing load and pulley, will move in response to stretching of the graft during pressurization. This upper assembly is also attached to an LVDT such that the changing length of the tube can be monitored to a precision of 5 microns. The operation of the system is quite simple in that the volume injected into the graft is monitored via the bellows LVDT. The pressure in the graft is monitored via the pressure transducer and the length of the graft is monitored via the sliding assembly LVDT. All data were acquired and recorded on a Macintosh-based LabVIEW ® data acquisition system. Once the system was calibrated, the tube was put into place and air removed via liquid injection. The motor was then started at a pulse rate of 70bpm while the injected volume, pressure, and graft length were simultaneously monitored and recorded. Once these data were captured for the 72bpm speed, the motor was adjusted to a speed of 400bpm and data were again collected. This process was repeated at 800, 1200, 1600, 2000 and, in some cases, 2700bpm. All data were analyzed for % radial compliance from 80 to 120mm Hg.
Stainless steel mandrils were produced and dipped into natural latex rubber to produce tubes of 6.50mm internal diameter. Two different wall thicknesses (0.030 and 0.015 inches) were used.
Results
To evaluate the internal compliance of a tube, the initial length and inside radius of the tube at atmospheric pressure must be measured. This was done using machinist’s pins. The initial volume of the rod that represents the inside lumen of the graft will be:
Vi lumen = π ri2 li
where ri = initial radius
li = initial length
If the tube is then inflated to some pressure p, a new set of dimensions results if the tube has any compliance. The new volume would be:
Vp = π rp2 lp
so that the change in volume from the initial state to the higher pressure state will be:
Vp – Vi = ∆V =π rp2 lp–π ri2 li
or (∆V /π) = rp2 lp-ri2 li
Since the initial dimensions and the change in volume are measurable, then:
(∆V /π) + ri2 li = rp2 lp
If the change in length is measurable, then:
((∆V / π) + ri2li) / lp = rp2 or rp = (((∆V / π) + ri2li) / lp)1/2
where rp is the new radius. For the purpose of convention, we normalize all results to a 100mm Hg increase in pressure so that:
( (rp-ri) / ri ) x (100 / ∆P) x 100 = % radial compliance
or ( (rp-ri) / ri ) / ∆P x 104 = % radial compliance
where ∆P is expressed in mm Hg. (Note: The formulas for dynamic compliance at subcritical speeds are identical to those for static determinations.) To determine the high speed properties of a vessel after the initial compliance is determined at 72bpm, one simply turns up the speed of the test while constantly monitoring the compliance of the vessel.
Table 1 presents the results from two samples of a 6.50mm I.D. tube with a wall thickness of 0.033 inches. Each sample was done in triplicate at the indicated speeds. Notice first that the sample-to-sample variation is quite low as indicated by the standard deviation. Also, results on the same sample from test to test are quite reproducible. As the speed of test increases up to 800bpm, the results are fairly consistent. When the testing speed reaches 1200bpm, there is a small decrease in compliance. This trend continues at 1600bpm where the radial compliance has dropped nearly 50%. As the speed is increased beyond this point, there appears to be a slight increase in the compliance.
Table 1
Percent Radial Compliance
Sample Test Rate
|
72 |
400
|
800
|
1200
|
1600
|
2000
|
2700
|
|
|
Dry 1A
|
3.55
|
3.36
|
3.29
|
3.18
|
1.96
|
2.41
|
|
|
Dry 1B
|
3.63
|
3.39
|
3.33
|
2.89
|
1.90
|
2.89
|
|
|
Dry 1C
|
3.62
|
3.46
|
3.29
|
2.89
|
2.08
|
2.38
|
|
|
Dry 2A
|
3.29
|
2.97
|
3.04
|
2.61
|
1.67
|
2.11
|
2.56
|
|
Dry 2B
|
3.35
|
3.17
|
3.07
|
2.67
|
1.93
|
2.87
|
2.81
|
|
Dry 2C
|
3.41
|
3.22
|
3.12
|
2.72
|
1.82
|
2.12
|
3.44
|
|
Average
|
3.48
|
3.26
|
3.19
|
2.83
|
1.89
|
2.46
|
2.94
|
|
Std. Dev.
|
0.14
|
0.18
|
0.13
|
0.21
|
0.14
|
0.35
|
0.45
|
|
%Std. Dev.
|
4.17
|
5.50
|
4.00
|
7.35
|
7.32
|
14.06
|
15.44
|
Table 2 is a compilation of the results from 6.5mm I.D. x 0.003″ wall samples that had been soaked for 48 hours in phosphate buffered saline. There is an increase in the compliance as the samples are soaked, probably due to hydration of the latex. The compliance starts to drop in the 1200 and 1600 range and recovers again at faster speeds. The Wet 48A sample at 2700, however, shows low compliance for reasons that are not clear at this time.
Table 2
Percent Radial Compliance
Sample Test Rate
|
72 |
400
|
800
|
1200
|
1600
|
2000
|
2700
|
|
|
Wet 48A
|
4.78
|
5.03
|
4.31
|
3.86
|
2.43
|
3.74
|
1.94
|
|
Wet 48B
|
4.79
|
5.16
|
4.18
|
2.49
|
2.59
|
3.95
|
2.78
|
|
Wet 48C
|
4.82
|
5.03
|
4.21
|
4.02
|
2.54
|
4.25
|
4.31
|
|
Average
|
4.80
|
5.07
|
4.23
|
3.46
|
2.52
|
3.98
|
3.01
|
|
Std. Dev.
|
0.02
|
0.08
|
0.07
|
0.84
|
0.08
|
0.26
|
1.20
|
|
%Std. Dev.
|
0.43
|
1.48
|
1.61
|
24.33
|
3.25
|
6.44
|
39.92
|
|
Wet 48S*
|
4.70
|
3.40
|
2.31
|
||||
|
*stretched 20%
|
Table 3 shows data obtained from samples similar to those in Tables 1 and 2. However, these samples were soaked for 96 hours in phosphate buffered saline. In general, it appears that the additional soaking has had little effect on the properties of the grafts.
Table 3
Percent Radial Compliance
Sample Test Rate
|
72 |
400
|
800
|
1200
|
1600
|
2000
|
2700
|
|
|
Wet 96A
|
4.82
|
5.00
|
4.22
|
4.21
|
2.63
|
3.05
|
3.21
|
|
Wet 96B
|
4.84
|
4.87
|
4.47
|
2.56
|
3.08
|
3.64
|
|
|
Wet 96C
|
4.84
|
4.87
|
|||||
|
Average
|
4.83
|
4.91
|
4.35
|
2.60
|
3.07
|
3.43
|
|
|
Std. Dev.
|
0.01
|
0.08
|
0.18
|
0.05
|
0.02
|
0.30
|
|
|
%Std. Dev.
|
0.24
|
1.53
|
4.07
|
1.91
|
0.69
|
8.88
|
Table 4 shows the results obtained from a 6.50mm I.D. x 0.015″ wall. As expected, the lower speed compliances are substantially higher than those obtained from the sample with the thicker wall. What is of interest, however, is the fact that this tube shows the same general results as the thicker-walled tubes with respect to a gradual reduction in compliance at a low point at 1600bpm with a recovery after that period. Unlike the thicker-walled tubes, an additional decrease in compliance seems to be occurring as speeds are increased up to 2700bpm.
Table 4
Percent Radial Compliance
Sample Test Rate
|
72 |
400
|
800
|
1200
|
1600
|
2000
|
2700
|
|
|
Dry 6.50, 0.015A
|
9.40
|
10.07
|
7.85
|
7.93
|
4.14
|
7.21
|
6.84
|
|
Dry 6.50, 0.015B
|
9.99
|
10.21
|
7.74
|
8.30
|
3.81
|
7.85
|
|
|
Dry 6.50, 0.015C
|
10.39
|
8.79
|
8.15
|
8.20
|
4.03
|
7.19
|
|
|
Average
|
9.93
|
9.69
|
7.91
|
8.14
|
3.99
|
7.42
|
|
|
Std. Dev.
|
0.50
|
0.78
|
0.21
|
0.19
|
0.17
|
0.38
|
|
|
%Std. Dev.
|
5.02
|
8.08
|
2.68
|
2.35
|
4.21
|
5.06
|
Conclusions
There are two possible explanations for the frequency dependent radial compliance observed in these studies. First, we must consider the presence of a harmonic oscillation that develops in the instrument or, second, that there is a complex relationship between frequency and radial compliance in these elastomeric tubes. Preliminary experimentation does not support the hypothesis of an harmonic oscillation, but further investigations are ongoing. On the other hand, if the second hypothesis is true, then it is critically important to have a comprehensive understanding of the mechanical properties of these and other tubes when loaded at different frequencies. That is, whether testing a tube or using it to hold another device, one must know the mechanical properties of that tube at the specific frequency and load range of the test.
References
1. Walden, R., L’Italien , G. J., Megerman , J., and Abbott, W. M., Matched Elastic Properties and Successful Arterial Grafting. Arch. Surg., 115, 1166-1169 (1980).
2. Kinley, C. E., and Marble, A. E., Compliance. J. Cardiovasc. Surg., 21, 163-170 (1980).
3. Clark , R. E., Apostolou, S., and Kardos, J. L., Mismatch of Mechanical Properties as a Cause of Arterial Prosthesis Thrombosis. Surg. Forum, 27, 208-217 (1976).
4. Gonza, E. R., Mason, W. F., Marble, A. E., and others, Necessity for Elastic Properties in Synthetic Arterial Grafts. Can. J. Surg., 17, 176-179 (1974).
5. Lyman, D. J., Fazzio, F. J., Voorhees, H., and others, Compliance as a Factor Effecting the Patency of Copolyurethane Vascular Grafts. J. Biomed. Master. Res., 12, 337-339 (1978).
6. Cengiz, M., Sauvage, L. R., Berger, K., Robel, S. B., Robel, V., Wu, H., Walker, M., Appleyard, R. F., and Wood, S. J., Effects of Compliance Alteration on Healing of a Porous Dacron Prosthesis in the Thoracic Aorta of the Dog. Surgery, Gynecology and Obstetrics, 58(2), 145 (1984).
7. Gow, B. S., and Taylor, G., Measurement of Viscoelastic Properties of Arteries in the Living Dog, Circulatory Research, 23, 111 (1968).
8. Gow, B. S., An Electrical Caliper for the Measurement of Pulsatile Arterial Diameter In Vivo. J. Appl. Physiol, 21, 1122 (1960).
9. Abbott, W. M., and Bouchier-Hayes, D. J., The Role of Mechanical Properties in Graft Design. In: Graft Materials in Vascular Surgery. Edited by H. Dardik. Miami: Symposia Specialists, Inc., 59 (1978).
10. Baird, R. N., Kidson, I. G., L’Italien, J. G., and Abbott, M. W., Dynamic Compliance of Arterial Grafts. Am. J. Physiol., 2, 568-572 (1977).
11. Bergel, D. H., The Static Elastic Properties of the Arterial Wall. J. Physiol. (London), 156, 445 (1961).
12. Bergel, D. H., The Dynamic Elastic Properties of the Arterial Wall. J. Physiol. (London), 156, 448 (1961).
13. Patel, D. J., De Freitan, F. M., Freenfield, J. R., and Fry, D. L., Relation of Radius to Pressure Along the Aorta in Living Dogs. J. Appl. Physiol, 18, 1111 (1963).
14. Paterson, L. H. Jensen, R. E. and Parnell, J., Mechanical Properties of Arteries In Vivo. Circulation Res., 8, 622 (1960).
15. Avolio, A. P., Fa-Quan, D., Wei-Qiang, L., Yao-Fei, L., Zhen-Dong, H., Lian-Fen, X., and 0’Rourke, M. F., Effects of Aging on Arterial Distensibility in Populations with High and Low Prevalence of Hypertension: Comparison Between Urban and Rural Communities in China. Circulation, 71(2), 202 (1985).
16. Tedgui, A. S., Levy, B.I., and Sebag, S. R., Instantaneous Blood Flow, Impedance and Elastic Properties Computed in Man from Aortic Pulse Waves. J. Theor. Biol., 101, 345-354 (1983).
17. Gotoh, F., Muramatsu, F., Fukuuchi, Y., Okaysu, H., Tanaka, K., Suzuki, N., and Kobari, M., Video Camera Method for Simultaneous Measurement of Blood Flow Velocity and Pial Vessel Diameter. J. Cereb. Blood Flow Metab.,
2, 421-428 (1982).
18. Assmann, R. and Henrich, H., A Video-Angiometer for Simultaneous and Continuous Measurement of Inner and Outer Vessel Diameters. Pflugers Arch., 376, 263-266 (1978).
19. Halpern, W., Mongeon, S. A., and Root, D. T., Stress, Tension and Myogenic Aspects of Small Isolated Extraparenchymal Rat Arteries. In: Smooth Muscle Contraction, edited by N.L. Stephens. New York : Dekker, 427-456 (1984).
20. Intaglietta, M. and Tompkins, W. R., On-Line Measurement of Microvascular Dimensions by Television Microscopy. J. Appl. Physiol., 32, 546-551 (1972).
21. Wiederhielm, C. A., Continuous Recording of Arteriolar Dimensions with a Television Microscope. J. Appl. Physiol., 18, 1041-1042 (1963).
22. Halpern, W., Osol, G., Coy, G. S., Mechanical Behavior of Pressurized In Vitro Prearteriolar Vessels Determined with a Video System. Annals of Biomedical Engineering, 12, 463-479 (1984).
23. Conti, J. C., Strope, E. R., Rohde, D. R. and Greisler, H. P., A New Technique to Determine Vascular Compliance In Vivo, National Heart Lung and Blood Institute Contractors Meeting. Louisville, KY, 1989.
24. Bozzi, R., Conti, J. C., Soldani, G., Spence, L., Strope, E., and Withrow, D., Relating the Frequency Dependent Radial Compliance to the Tensile Modulus of Polyurethane and Latex Vascular Grafts. Transactions 5th World Biomaterials Congress, 434, U2 (1996).



